Given strings A, B and C, this approach finds whether C is formed by the interleaving of strings A and B.
Let us assume, A = "ab", B="cd" and C="acbd", then we can say that C is formed by interleaving of strings A and B.
But if A = "ab", B="cd" and C="adbc", then we can say that C cannot be formed by interleaving of strings A and B. This is due to the fact that in C, the character 'd' has come before C.
if A = "ab", B="cd" and C="abcdx", then also we can say that C cannot be formed by interleaving of strings A and B. This is due to the fact that in C has a character which is not in A or B.
Now, let us assume C is a 50 character string and A is a 20 character string and B is 30 character string. If C can be formed by interleaving of A and B, then any prefix of C can be formed by interleaving some prefix of A and some prefix of B.
Assume A(n), B(n), C(n) denotes the prefix of A with n characters, prefix of B with first n characters and prefix of C with first n characters.
Assume C(20) can be formed by interleaving of some prefix of A and B. We know that C(20) can be formed by some combining characters of A(0),B(20) or A(1), B(19) or A(2),B(18),.................., A(18), B(2) or A(19), B(1) or A(20),B(0) etc. There can be 21 such combinations and characters interleaved from some of these combinations result in C(20).
Now let us check if C(21) can be formed by interleaving some prefixes of A or B. It will be possible if A(i),B(j) gives C(20) (i.e. i + j == 20) and either A[i+1] is same as the 21st character of C, or B[j+1] is the 21st character of C. Assume A[i+1] is same as 21st character of C, then we can say that A(i+1),B(j) can be interleaved to form C(21).
In dynamic programming, we use the stored results previously computed. For checking C(21), we check for all combinations of A(i),B(j) where i + j = 20 and 0 <= i,j <= 20 and all combinations are already computed.
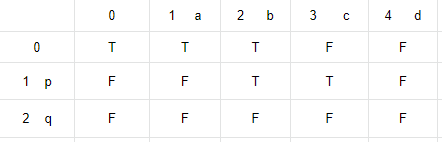
Now let us look at the below example. We will see how we check if C="abpcqd" is interleaving of A="abcd" and B="pq".
We initialize a two dimensional matrix of M size (2+1)x(4+1) and set all the cells to false. If M[i][j] is true, then first 'i' chars of B and first 'j' chars from A can be interleaved to get the string with first 'i + j' chars from C.
We have taken a matrix of 3x5 dimension. Row 0 finds how much of C can be formed by interleaving only chars from A, and taking 0 chars from B. M[0][0] = T (true) as null string from C can be formed by taking 0 chars from A and B.
Now let us fill all the values for Row. Row[0][1] = T, because C(1) can be formed by A(1) alone.
Row[0][2] will be T again, as C(2) can be formed by A(2) alone. Row[0][3], Row[0,4] remain F as C(3) and hence C(4) also cannot be formed from A(3) and A(4) alone.
Row[1][0] will remain false as C(1) cannot be formed from B(1) alone. Similarly Row[2][0] remains false.
Now let us start modifying Row[1] values. M[1][1] will remain F. Because though M[0][1] is T, C[1+1 - 0] = C[1] != B[0]. Row[1][2] will be T, as M[0][2] is true (that means C(3) is containing 'ab') and C[1+2 - 1]=C[2]==B[1]. Row[1][2] = T means, C(3) can be formed by interleaving B(1) and A(2). Now Row[1] is only for taking 1 char B[0] from B. As Row[1][2] is T, so for subsequent comparison on this row, we will use chars from A only. Row[1][3] will be T as Row[1][2] is T and C[3] == A[2]. Row[1][4] will remain F, as though Row[1][3] = True, but C[4]!=A[3]
Similarly, we check Row[2]. Row[2][3] will be T as Row[1][3] == T and C[4] == B[2].
Row[2,4] will be T as Row[2][3] == T and C[5] == A[3].
Python implementation of above algorithm is given below:
''' Given A, B, C, find whether C is formed by the interleaving of A and B. Example: 1: A = "ab" B = "cd" C = "acdb" Here, C can be formed by the interleaving of A and B 2: A = "ab" B = "cd" C = "adbc" Here, C cannot be formed by the interleaving of A and B as 'd' and 'c' are coming out of orders in C. 2: A = "ab" B = "cd" C = "acd" Here, C cannot be formed by the interleaving of A and B as 'b' is missing in C ''' def is_interleaving(first, second, interleaved): if len(first) + len(second) != len(interleaved): return False; if len(first) == 0: return second == interleaved if len(second) == 0: return first == interleaved # interleaved must start with 0th char of first or second string if not first[0] == interleaved[0] and not second[0] == interleaved[0]: return False i = len(first) + 1 j = len(second) + 1 k = 0 matrix = [] while k < j: matrix.append([False] * i) k += 1 # 0 char from first, 0 char from second is equal 0 # char from interleaved matrix[0][0] = True # Now check how much of interleaved string can be formed # by using 0 char from second and all others from first k = 1 while k < i: if matrix[0][k - 1] and (first[k - 1] == interleaved[k - 1]): matrix[0][k] = True else: break k += 1 # Now check how much of interleaved string can be formed # by using 0 char from first and all others from second k = 1 while k < j: if matrix[0][0] and (second[k - 1] == interleaved[k - 1]): matrix[k][0] = True else: break k += 1 # Now we successively find out if interleaved[:n+m] can be formed # by interleaving first n chars from first and m chars from second # m varies from 1 to len(first) # n varies from 1 to len(second) # When we are on xth row of the matrix, we are actually trying to # check if (x - 1) chars from second string have been already seen, # and for that to happen, x - 2 chars have to be already present # in some prefix of interleaved. k = 1 ksecond_matched = False while k < j: #checking for a prefix of interleaved which can be formed #with k chars from second l = 1 ksecond_matched = matrix[k][0] while l < i: if not ksecond_matched: if matrix[k-1][l] and interleaved[k + l - 1] == second[k-1]: matrix[k][l] = True ksecond_matched = True else: # we have already matched k chars from second, check if a prefix # of length k + x can be obtained which is interleaved with # first k and x chars from second and first respectively if matrix[k][l - 1] and interleaved[k + l - 1] == first[l-1]: matrix[k][l] = True l += 1 k += 1 return matrix[j - 1][i - 1] test_data = [['a', 'b', 'ab', True], ['ab', '', 'ab', True], ['abc', 'd', 'abcd', True], ['ab', 'cd', 'abcd', True], ['ab', 'cd', 'abcde', False], ['ab', 'cde', 'aced', False], ['ab', 'cde', 'abcd' , False], ['ab', 'cde', 'aecdb', False], ['ab', 'cde', 'abcde', True]] for row in test_data: if is_interleaving(row[0], row[1], row[2]) != row[3]: print '!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!Failed for ', row else: print 'Passed for', row
#include <string> #include <iostream> #include <vector> #include <algorithm> #include <tuple> using std::string; using std::vector; using std::cout; using std::endl; using std::tuple; using std::make_tuple; using std::get; bool is_interleaving(const string& first, const string& second, const string& interleaved) { if (first.size() + second.size() != interleaved.size()) { return false; } if (first.size() == 0) { return second == interleaved; } if (second.size() == 0) { return first == interleaved; } if (second[0] != interleaved[0] && first[0] != interleaved[0]) { return false; } int i = first.size() + 1; int j = second.size() + 1; int k = 0; vector<vector<bool> > matrix; while (k++ < j) { matrix.push_back(vector<bool>(false,i)); } // 0 char from first, 0 char from second is equal 0 // char from interleaved matrix[0][0] = true; // Now check how much of interleaved string can be formed // by using 0 char from second and all others from first k = 1; while (k < i) { if (matrix[0][k - 1] && (first[k - 1] == interleaved[k - 1])) matrix[0][k] = true; else break; k++; } // Now check how much of interleaved string can be formed // by using 0 char from first and all others from second k = 1; while (k < j) { if (matrix[0][0] && (second[k - 1] == interleaved[k - 1])) matrix[k][0] = true; else break; k++; } // Now we successively find out if interleaved[:n+m] can be formed // by interleaving first n chars from first and m chars from second // m varies from 1 to len(first) // n varies from 1 to len(second) // When we are on xth row of the matrix, we are actually trying to // check if (x - 1) chars from second string have been already seen, // and for that to happen, x - 2 chars have to be already present // in some prefix of interleaved. k = 1; int l = 0; bool ksecond_matched = false; while (k < j) { //checking for a prefix of interleaved which can be formed //with k chars from second l = 1; ksecond_matched = matrix[k][0]; while (l < i) { if (!ksecond_matched) { if (matrix[k-1][l] && interleaved[k + l - 1] == second[k-1]) { matrix[k][l] = true; ksecond_matched = true; } } else { // we have already matched k chars from second, check if a prefix // of length k + x can be obtained which is interleaved with // first k and x chars from second and first respectively if (matrix[k][l - 1] && interleaved[k + l - 1] == first[l-1]) matrix[k][l] = true; } l++; } k++; } return matrix[j - 1][i - 1]; } // test run int main() { cout << "Running some tests for the implementation" << endl; vector<tuple<string, string, string, bool> > inputs; inputs.push_back(make_tuple("a", "b", "ab", true)); inputs.push_back(make_tuple("ab", "", "ab", true)); inputs.push_back(make_tuple("abc", "d", "abcd", true)); inputs.push_back(make_tuple("abc", "d", "acbd", false)); inputs.push_back(make_tuple("a", "bc", "ab", false)); inputs.push_back(make_tuple("ab", "bc", "abbc", true)); inputs.push_back(make_tuple("ab", "bc", "acbb", false)); inputs.push_back(make_tuple("ac", "bc", "abbc", false)); for_each(inputs.begin(), inputs.end(), [](tuple<string, string, string, bool>& data) { cout << "Cheking for str1 = " << get<0>(data) << " str2 = " << get<1>(data) << " interleaved = " << get<2>(data) << " expected=" << std::boolalpha << get<3>(data); if (is_interleaving(get<0>(data), get<1>(data), get<2>(data)) != get<3>(data)){ cout << " --> Failed " << endl; } else { cout << " --> Success " << endl; } }); }